electromagnetism - How can radiation be a transverse wave? Does light really resemble a rope? How can a 3D field be a medium for non-spatial 1D waves? Need mental model

I understand longitudinal waves. For example, I've got a clear mental modal of air waves: a slice of air becomes overcompressed, then the slice next to it becomes overcompressed and the first slice becomes undercompressed. The air itself does not travel, but the overcompressed and undercompressed regions do travel, and that's a wave:

I also understand transverse waves. For example, ripples on the water surface. The surface is a 2D field, the ripples oscillate in the third dimension (thus, water waves are a 3D structure). But transverse waves can also exist on a true 2D plane without the third dimension, here's an example:

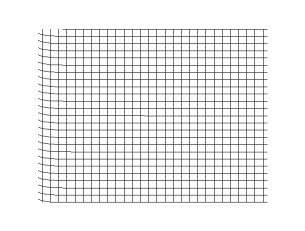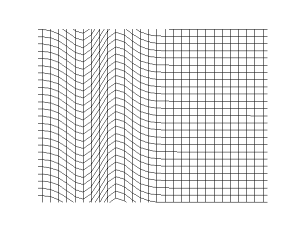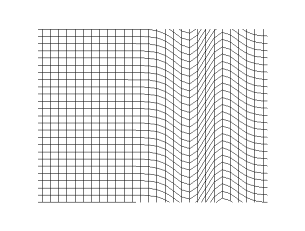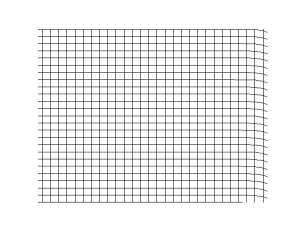
But as for radiation waves, I fail to find a mental model for them. The closest one seems to be a rope: if you wobble a wire holding it by its end, it will oscillate in a sine-like pattern. I see it as a 1D field oscillating in a second dimension (thus, a wobbling wire is a 2D structure):

(these are an electric and a magnetic wave, the two are different waves that travel in different fields, they just happen to always appear in pairs)

Note how B and E arrows on the above animation demonstrate how the field wobbles without travelling.

I also know that light waves can be radially polarized and they are depicted as a spiral. This also corresponds to the wobbling rope metaphor:

But the electric and magnetic fields are 3D, yet light is not spatial like air is. When a light wave travels through its field, it looks like it occupies an 1D line of a 3D field, and this line wobbles either in one extra dimension (linear polarization) or in two extra dimensions (radial polarization).

This does not make sense to me. A wobbling rope could be a great depiction of a light wave, but it's a poor metaphor: the medium of a rope wave is a rope itself, and a rope is kinda 1D (in a sense that it's a line). But the medium for a light wave is an electirc field, and the field is 3D, it's spatial.

So how can 3D spatial field be a medium for string-like non-spatial waves? And is there a human-world analogy for this phenomenon?

PS This must have something to do with the wave-particle duality, but I don't understand that either.

UPD I no longer understand what is a transverse wave and what is a longitudinal wave.

Water ripples are transverse, right? The waves spread parallel to the water surface but the oscillations happen perpendicular to the water surface. Thus, transverse.

But if you look at the flat water surface from above (from the third dimension), it will not look like the transverse animation. It will look exactly the longitudinal one! What happens here?

Do light waves appear longitudinal when observed from a fourth dimension? Mind: blown.