The question arose when my physics teacher taught me geometrical optics and told that the phenomenon of both refraction and reflection occur by change in medium. First of all, I'm not sure about the above and would like a bit clarity on this. And the main doubt emerges when I make the scenario given in the picture below:

Sorry for my bad drawing, but as you can see, my question is that what happens when I make the ray incident at (a)almost equal to critical angle (i limiting to ic) and (b) at exactly ic . For the (b) part I saw this (Does light reflect if incident at exactly the critical angle?) question. But the above concept my sir taught kinda contradicts for part (a). I have given the options which should occur in the picture, (a) and (b), with (a) being wha my sir said and (b) being the other option.
If (a) is occurring, which should occur according to my teacher, then it contradicts principle of reversibility, or actually common sense, since the reverse ray won't even touch the surface, and if (b) occurs then it contradicts what my teacher taught me.
So is the teacher wrong? Where lies the fallacy?
Answer
In general, reflection and refraction happen when light passes from one medium to another. You can see this if you see your own reflection in a window. Now, as a light ray approaches the critical angle, not only does the refracted ray get closer to the surface, but the amount of light transmitted gets less and less. At the critical angle, the refracted ray would be along the surface, but the amount of light is zero.
The graph below shows how much light is reflected at various angles of incidence. The graph on the right shows your situation of a ray of light moving from a dense medium to a lighter medium (glass to air, for example). At the critical angle, 100% of light is reflected, leaving none to propagate along the surface.
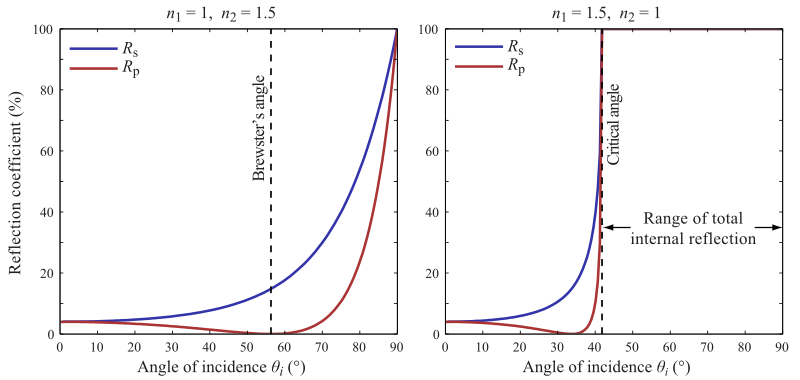
from http://en.wikipedia.org/wiki/Fresnel_equations
No comments:
Post a Comment